大门|大门-CS-2005 |问题90

下面是偏序集[{a，b，c，d，e}的哈斯图，≤] 装束是

（A） 不是格子 （B） 一个格但不是分配格 （C） 分配格但不是布尔代数 （D） 布尔代数 答复: （B） 说明：

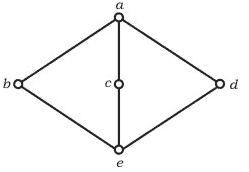
It is a lattice but not a distributive lattice.

Table for Join Operation of above Hesse diagram

V |a b c d e
________________
a |a a a a a
b |a b a a b
c |a a c a c
d |a a a d d
e |a b c d e

Table for Meet Operation of above Hesse diagram

^ |a b c d e
_______________
a |a b c d e
b |b b e e e
c |c e c e e
d |d e e d e
e |e e e e e

Therefore for any two element p, q in the lattice (A,<=)

p <= p V q ; p^q <= p

This satisfies for all element (a,b,c,d,e).

which has 'a' as unique least upper bound and 'e' as unique 
greatest lower bound.

The given lattice doesn't obey distributive law, so it is 
not distributive lattice,

Note that for b,c,d we have distributive law

b^(cVd) = (b^c) V (b^d). From the diagram / tables given above 
we can verify as follows,

(i) L.H.S. = b ^ (c V d) = b ^ a = b

(ii) R.H.S. = (b^c) V (b^d) = e v e = e

b != e which contradict the distributive law. 
Hence it is not distributive lattice.

so, option (B) is correct. 

这个问题的小测验