给定两个坐标(x1,y1)和(x2,y2),以及m和n,求出将连接线(x1,y1)和(x2,y2)以m:n的比率分开的坐标
null
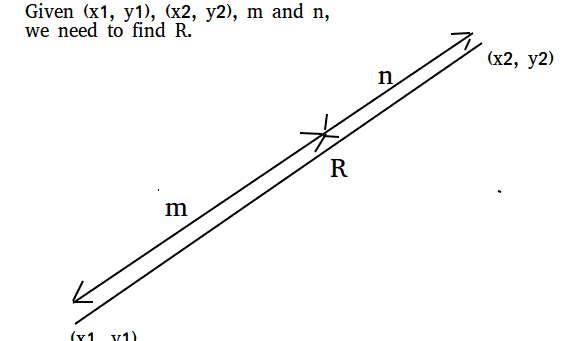
例如:
Input : x1 = 1, y1 = 0, x2 = 2 y2 = 5,
m = 1, n = 1
Output : (1.5, 2.5)
Explanation: co-ordinates (1.5, 2.5)
divides the line in ratio 1 : 1
Input : x1 = 2, y1 = 4, x2 = 4, y2 = 6,
m = 2, n = 3
Output : (2.8, 4.8)
Explanation: (2.8, 4.8) divides the line
in the ratio 2:3
截面公式告诉我们将给定线段分成两部分的点的坐标,其长度为m:n
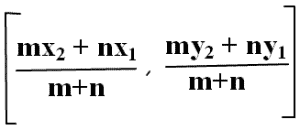
C++
// CPP program to find point that divides // given line in given ratio. #include <iostream> using namespace std; // Function to find the section of the line void section( double x1, double x2, double y1, double y2, double m, double n) { // Applying section formula double x = ((n * x1) + (m * x2)) / (m + n); double y = ((n * y1) + (m * y2)) / (m + n); // Printing result cout << "(" << x << ", " ; cout << y << ")" << endl; } // Driver code int main() { double x1 = 2, x2 = 4, y1 = 4, y2 = 6, m = 2, n = 3; section(x1, x2, y1, y2, m, n); return 0; } |
JAVA
// Java program to find point that divides // given line in given ratio. import java.io.*; class sections { static void section( double x1, double x2, double y1, double y2, double m, double n) { // Applying section formula double x = ((n * x1) + (m * x2)) / (m + n); double y = ((n * y1) + (m * y2)) / (m + n); // Printing result System.out.println( "(" + x + ", " + y + ")" ); } public static void main(String[] args) { double x1 = 2 , x2 = 4 , y1 = 4 , y2 = 6 , m = 2 , n = 3 ; section(x1, x2, y1, y2, m, n); } } |
python
# Python program to find point that divides # given line in given ratio. def section(x1, x2, y1, y2, m, n): # Applying section formula x = ( float )((n * x1) + (m * x2)) / (m + n) y = ( float )((n * y1) + (m * y2)) / (m + n) # Printing result print (x, y) x1 = 2 x2 = 4 y1 = 4 y2 = 6 m = 2 n = 3 section(x1, x2, y1, y2, m, n) |
C#
// C# program to find point that divides // given line in given ratio. using System; class GFG { static void section( double x1, double x2, double y1, double y2, double m, double n) { // Applying section formula double x = ((n * x1) + (m * x2)) / (m + n); double y = ((n * y1) + (m * y2)) / (m + n); // Printing result Console.WriteLine( "(" + x + ", " + y + ")" ); } // Driver code public static void Main() { double x1 = 2, x2 = 4, y1 = 4, y2 = 6, m = 2, n = 3; section(x1, x2, y1, y2, m, n); } } // This code is contributed by vt_m. |
PHP
<?php // PHP program to find point that // divides given line in given ratio. // Function to find the // section of the line function section( $x1 , $x2 , $y1 , $y2 , $m , $n ) { // Applying section formula $x = (( $n * $x1 ) + ( $m * $x2 )) / ( $m + $n ); $y = (( $n * $y1 ) + ( $m * $y2 )) / ( $m + $n ); // Printing result echo ( "(" . $x . ", " ); echo ( $y . ")" ); } // Driver code $x1 = 2; $x2 = 4; $y1 = 4; $y2 = 6; $m = 2; $n = 3; section( $x1 , $x2 , $y1 , $y2 , $m , $n ); // This code is contributed by Ajit. ?> |
Javascript
<script> // JavaScript program to find point that divides // given line in given ratio function section(x1, x2, y1, y2, m, n) { // Applying section formula let x = ((n * x1) + (m * x2)) / (m + n); let y = ((n * y1) + (m * y2)) / (m + n); // Printing result document.write( "(" + x + ", " + y + ")" ); } // Driver Code let x1 = 2, x2 = 4, y1 = 4, y2 = 6, m = 2, n = 3; section(x1, x2, y1, y2, m, n) // This code is contributed by avijitmondal1998. </script> |
输出:
(2.8, 4.8)
这是怎么回事?
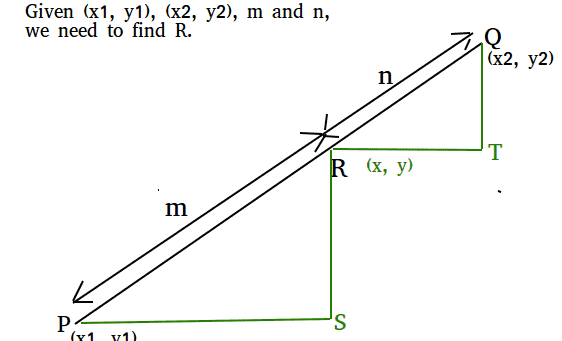
From our diagram, we can see, PS = x – x1 and RT = x2 – x We are given, PR/QR = m/n Using similarity, we can write RS/QT = PS/RT = PR/QR Therefore, we can write PS/RR = m/n (x - x1) / (x2 - x) = m/n From above, we get x = (mx2 + nx1) / (m + n) Similarly, we can solve for y.
参考资料: http://doubleroot.in/lessons/coordinate-geometry-basics/section-formula/#.WjYXQvbhU8o
© 版权声明
文章版权归作者所有,未经允许请勿转载。
THE END


![关于”PostgreSQL错误:关系[表]不存在“问题的原因和解决方案-yiteyi-C++库](https://www.yiteyi.com/wp-content/themes/zibll/img/thumbnail.svg)



