找出给定字符串中可以形成多少回文子序列(不一定是不同的)。请注意,空字符串不被视为回文。 例如:
null
Input : str = "abcd"Output : 4Explanation :- palindromic subsequence are : "a" ,"b", "c" ,"d" Input : str = "aab"Output : 4Explanation :- palindromic subsequence are :"a", "a", "b", "aa"Input : str = "aaaa"Output : 15
上述问题可以递归定义。
Initial Values : i= 0, j= n-1;CountPS(i,j)// Every single character of a string is a palindrome // subsequence if i == j return 1 // palindrome of length 1// If first and last characters are same, then we // consider it as palindrome subsequence and check// for the rest subsequence (i+1, j), (i, j-1)Else if (str[i] == str[j)] return countPS(i+1, j) + countPS(i, j-1) + 1;else // check for rest sub-sequence and remove common // palindromic subsequences as they are counted // twice when we do countPS(i+1, j) + countPS(i,j-1) return countPS(i+1, j) + countPS(i, j-1) - countPS(i+1, j-1)
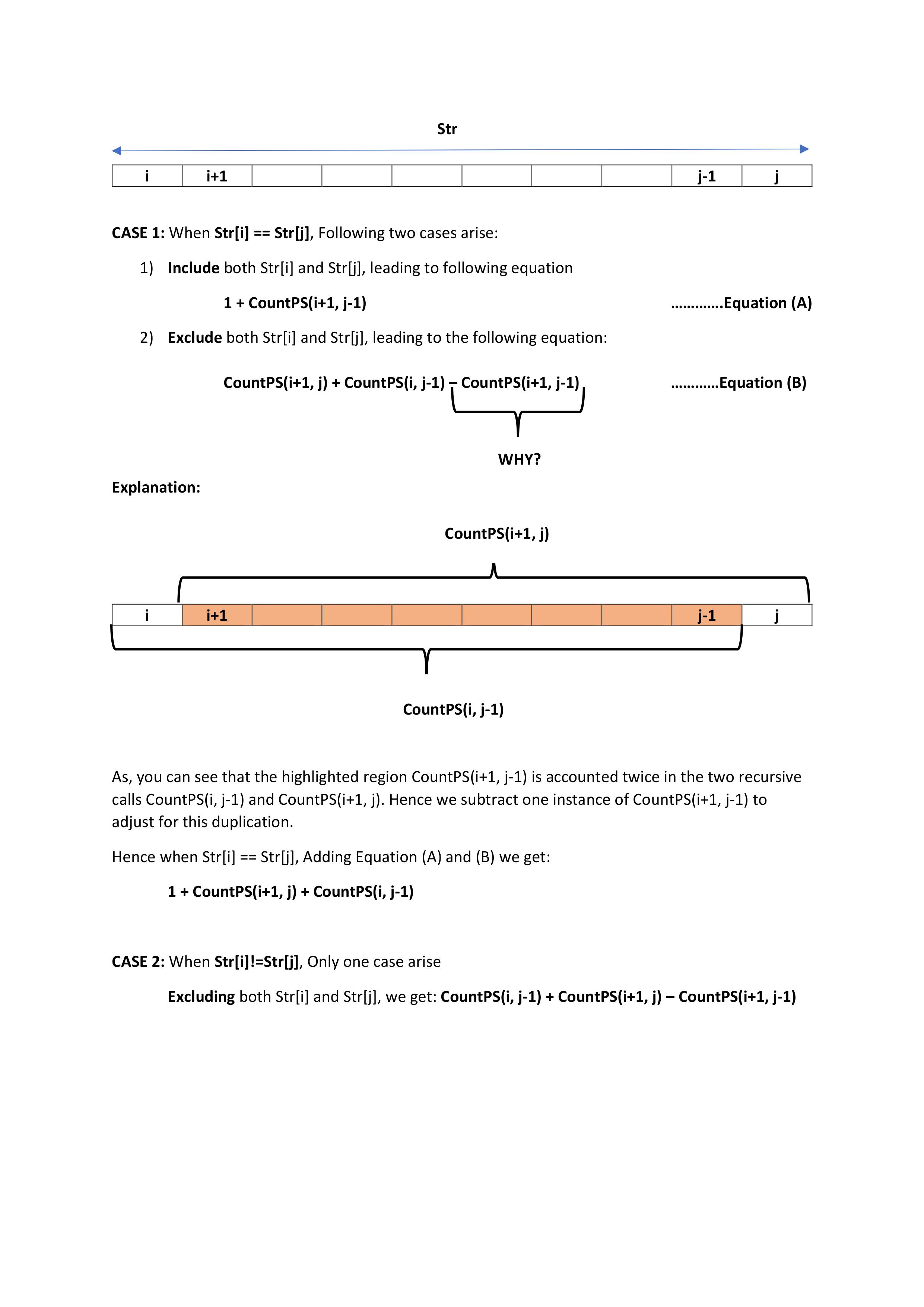
如果我们画出上述递归解的递归树,我们可以观察到 重叠子问题 由于这个问题有重叠的子问题,我们可以使用动态规划有效地解决它。下面是基于动态规划的解决方案。
C++
// Counts Palindromic Subsequence in a given String #include <cstring> #include <iostream> using namespace std; // Function return the total palindromic subsequence int countPS(string str) { int N = str.length(); // create a 2D array to store the count of palindromic // subsequence int cps[N + 1][N + 1]; memset (cps, 0, sizeof (cps)); // palindromic subsequence of length 1 for ( int i = 0; i < N; i++) cps[i][i] = 1; // check subsequence of length L is palindrome or not for ( int L = 2; L <= N; L++) { for ( int i = 0; i <= N-L; i++) { int k = L + i - 1; if (str[i] == str[k]) cps[i][k] = cps[i][k - 1] + cps[i + 1][k] + 1; else cps[i][k] = cps[i][k - 1] + cps[i + 1][k] - cps[i + 1][k - 1]; } } // return total palindromic subsequence return cps[0][N - 1]; } // Driver program int main() { string str = "abcb" ; cout << "Total palindromic subsequence are : " << countPS(str) << endl; return 0; } |
JAVA
// Java code to Count Palindromic Subsequence // in a given String public class GFG { // Function return the total palindromic // subsequence static int countPS(String str) { int N = str.length(); // create a 2D array to store the count // of palindromic subsequence int [][] cps = new int [N][N]; // palindromic subsequence of length 1 for ( int i = 0 ; i < N; i++) cps[i][i] = 1 ; // check subsequence of length L is // palindrome or not for ( int L = 2 ; L <= N; L++) { for ( int i = 0 ; i <= N-L; i++) { int k = L + i - 1 ; if (str.charAt(i) == str.charAt(k)) { cps[i][k] = cps[i][k - 1 ] + cps[i + 1 ][k] + 1 ; } else { cps[i][k] = cps[i][k - 1 ] + cps[i + 1 ][k] - cps[i + 1 ][k - 1 ]; } } } // return total palindromic subsequence return cps[ 0 ][N - 1 ]; } // Driver program public static void main(String args[]) { String str = "abcb" ; System.out.println( "Total palindromic " + "subsequence are : " + countPS(str)); } } // This code is contributed by Sumit Ghosh |
Python3
# Python3 code to Count Palindromic # Subsequence in a given String # Function return the total # palindromic subsequence def countPS( str ): N = len ( str ) # Create a 2D array to store the count # of palindromic subsequence cps = [[ 0 for i in range (N + 2 )] for j in range (N + 2 )] # palindromic subsequence of length 1 for i in range (N): cps[i][i] = 1 # check subsequence of length L # is palindrome or not for L in range ( 2 , N + 1 ): for i in range (N): k = L + i - 1 if (k < N): if ( str [i] = = str [k]): cps[i][k] = (cps[i][k - 1 ] + cps[i + 1 ][k] + 1 ) else : cps[i][k] = (cps[i][k - 1 ] + cps[i + 1 ][k] - cps[i + 1 ][k - 1 ]) # return total palindromic subsequence return cps[ 0 ][N - 1 ] # Driver program str = "abcb" print ( "Total palindromic subsequence are : " , countPS( str )) # This code is contributed by Anant Agarwal. |
C#
// C# code to Count Palindromic Subsequence // Subsequence in a given String using System; class GFG { // Function return the total // palindromic subsequence static int countPS( string str) { int N = str.Length; // create a 2D array to store the // count of palindromic subsequence int [, ] cps = new int [N + 1, N + 1]; // palindromic subsequence // of length 1 for ( int i = 0; i < N; i++) cps[i, i] = 1; // check subsequence of length // L is palindrome or not for ( int L = 2; L <= N; L++) { for ( int i = 0; i <= N-L; i++) { int k = L + i - 1; if (k < N) { if (str[i] == str[k]) cps[i, k] = cps[i, k - 1] + cps[i + 1, k] + 1; else cps[i, k] = cps[i, k - 1] + cps[i + 1, k] - cps[i + 1, k - 1]; } } } // return total palindromic // subsequence return cps[0, N - 1]; } // Driver Code public static void Main() { string str = "abcb" ; Console.Write( "Total palindromic " + "subsequence are : " + countPS(str)); } } // This code is contributed by nitin mittal. |
PHP
<?php // Counts Palindromic Subsequence in // a given String // Function return the total // palindromic subsequence function countPS( $str ) { $N = strlen ( $str ); // create a 2D array to store the // count of palindromic subsequence $cps = array_fill (0, $N + 1, array_fill (0, $N + 1, NULL)); // palindromic subsequence of length 1 for ( $i = 0; $i < $N ; $i ++) $cps [ $i ][ $i ] = 1; // check subsequence of length L // is palindrome or not for ( $L = 2; $L <= $N ; $L ++) { for ( $i = 0; $i <= $N - $L ; $i ++) { $k = $L + $i - 1; if ( $str [ $i ] == $str [ $k ]) $cps [ $i ][ $k ] = $cps [ $i ][ $k - 1] + $cps [ $i + 1][ $k ] + 1; else $cps [ $i ][ $k ] = $cps [ $i ][ $k - 1] + $cps [ $i + 1][ $k ] - $cps [ $i + 1][ $k - 1]; } } // return total palindromic subsequence return $cps [0][ $N - 1]; } // Driver Code $str = "abcb" ; echo "Total palindromic subsequence are : " . countPS( $str ) . "" ; // This code is contributed by ita_c ?> |
Javascript
<script> // Javascript code to Count Palindromic Subsequence // in a given String // Function return the total palindromic // subsequence function countPS(str) { let N = str.length; // create a 2D array to store the count // of palindromic subsequence let cps = new Array(N); for (let i=0;i<N;i++) { cps[i]= new Array(N); for (let j=0;j<N;j++) { cps[i][j]=0; } } // palindromic subsequence of length 1 for (let i = 0; i < N; i++) cps[i][i] = 1; // check subsequence of length L is // palindrome or not for (let L = 2; L <= N; L++) { for (let i = 0; i <= N-L; i++) { let k = L + i - 1; if (str[i] == str[k]) { cps[i][k] = cps[i][k - 1] + cps[i + 1][k] + 1; } else { cps[i][k] = cps[i][k - 1] + cps[i + 1][k] - cps[i + 1][k - 1]; } } } // return total palindromic subsequence return cps[0][N - 1]; } // Driver program let str = "abcb" ; document.write( "Total palindromic " + "subsequence are : " + countPS(str)); // This code is contributed by avanitrachhadiya2155 </script> |
输出:
Total palindromic subsequence are : 6
时间复杂性: O(N) 2. )
另一种方法: (使用递归)
C++
// C++ program to counts Palindromic Subsequence // in a given String using recursion #include <bits/stdc++.h> using namespace std; int n, dp[1000][1000]; string str = "abcb" ; // Function return the total // palindromic subsequence int countPS( int i, int j) { if (i > j) return 0; if (dp[i][j] != -1) return dp[i][j]; if (i == j) return dp[i][j] = 1; else if (str[i] == str[j]) return dp[i][j] = countPS(i + 1, j) + countPS(i, j - 1) + 1; else return dp[i][j] = countPS(i + 1, j) + countPS(i, j - 1) - countPS(i + 1, j - 1); } // Driver code int main() { memset (dp, -1, sizeof (dp)); n = str.size(); cout << "Total palindromic subsequence are : " << countPS(0, n - 1) << endl; return 0; } // this code is contributed by Kushdeep Mittal |
JAVA
// Java program to counts Palindromic Subsequence // in a given String using recursion class GFG { static int n; static int [][] dp = new int [ 1000 ][ 1000 ]; static String str = "abcb" ; // Function return the total // palindromic subsequence static int countPS( int i, int j) { if (i > j) return 0 ; if (dp[i][j] != - 1 ) return dp[i][j]; if (i == j) return dp[i][j] = 1 ; else if (str.charAt(i) == str.charAt(j)) return dp[i][j] = countPS(i + 1 , j) + countPS(i, j - 1 ) + 1 ; else return dp[i][j] = countPS(i + 1 , j) + countPS(i, j - 1 ) - countPS(i + 1 , j - 1 ); } // Driver code public static void main(String[] args) { for ( int i = 0 ; i < 1000 ; i++) for ( int j = 0 ; j < 1000 ; j++) dp[i][j] = - 1 ; n = str.length(); System.out.println( "Total palindromic subsequence" + "are : " + countPS( 0 , n - 1 )); } } // This code is contributed by Ryuga |
Python 3
# Python 3 program to counts Palindromic # Subsequence in a given String using recursion str = "abcb" # Function return the total # palindromic subsequence def countPS(i, j): if (i > j): return 0 if (dp[i][j] ! = - 1 ): return dp[i][j] if (i = = j): dp[i][j] = 1 return dp[i][j] else if ( str [i] = = str [j]): dp[i][j] = (countPS(i + 1 , j) + countPS(i, j - 1 ) + 1 ) return dp[i][j] else : dp[i][j] = (countPS(i + 1 , j) + countPS(i, j - 1 ) - countPS(i + 1 , j - 1 )) return dp[i][j] # Driver code if __name__ = = "__main__" : dp = [[ - 1 for x in range ( 1000 )] for y in range ( 1000 )] n = len ( str ) print ( "Total palindromic subsequence are :" , countPS( 0 , n - 1 )) # This code is contributed by ita_c |
C#
// C# program to counts Palindromic Subsequence // in a given String using recursion using System; class GFG { static int n; static int [, ] dp = new int [1000, 1000]; static string str = "abcb" ; // Function return the total // palindromic subsequence static int countPS( int i, int j) { if (i > j) return 0; if (dp[i, j] != -1) return dp[i, j]; if (i == j) return dp[i, j] = 1; else if (str[i] == str[j]) return dp[i, j] = countPS(i + 1, j) + countPS(i, j - 1) + 1; else return dp[i, j] = countPS(i + 1, j) + countPS(i, j - 1) - countPS(i + 1, j - 1); } // Driver code static void Main() { for ( int i = 0; i < 1000; i++) for ( int j = 0; j < 1000; j++) dp[i, j] = -1; n = str.Length; Console.Write( "Total palindromic subsequence" + "are : " + countPS(0, n - 1)); } } // This code is contributed by DrRoot_ |
PHP
<?php // PHP program to counts Palindromic Subsequence // in a given String using recursion $dp = array_fill (0, 100, array_fill (0, 1000, -1)); $str = "abcb" ; $n = strlen ( $str ); // Function return the total // palindromic subsequence function countPS( $i , $j ) { global $str , $dp , $n ; if ( $i > $j ) return 0; if ( $dp [ $i ][ $j ] != -1) return $dp [ $i ][ $j ]; if ( $i == $j ) return $dp [ $i ][ $j ] = 1; else if ( $str [ $i ] == $str [ $j ]) return $dp [ $i ][ $j ] = countPS( $i + 1, $j ) + countPS( $i , $j - 1) + 1; else return $dp [ $i ][ $j ] = countPS( $i + 1, $j ) + countPS( $i , $j - 1) - countPS( $i + 1, $j - 1); } // Driver code echo "Total palindromic subsequence are : " . countPS(0, $n - 1); // This code is contributed by mits ?> |
Javascript
<script> // Javascript program to counts Palindromic Subsequence // in a given String using recursion let n; let dp= new Array(1000); for (let i=0;i<1000;i++) { dp[i]= new Array(1000); for (let j=0;j<1000;j++) { dp[i][j]=-1; } } let str = "abcb" ; // Function return the total // palindromic subsequence function countPS(i,j) { if (i > j) return 0; if (dp[i][j] != -1) return dp[i][j]; if (i == j) return dp[i][j] = 1; else if (str[i] == str[j]) return dp[i][j] = countPS(i + 1, j) + countPS(i, j - 1) + 1; else return dp[i][j] = countPS(i + 1, j) + countPS(i, j - 1) - countPS(i + 1, j - 1); } // Driver code n = str.length; document.write( "Total palindromic subsequence" + "are : " + countPS(0, n - 1)); // This code is contributed by rag2127 </script> |
输出:
Total palindromic subsequence are : 6
本文由 西山东星(品图) .如果你喜欢GeekSforgek,并想贡献自己的力量,你也可以使用 写极客。组织 或者把你的文章寄去评论-team@geeksforgeeks.org.看到你的文章出现在Geeksforgeks主页上,并帮助其他极客。 如果您发现任何不正确的地方,或者您想分享有关上述主题的更多信息,请写下评论。
© 版权声明
文章版权归作者所有,未经允许请勿转载。
THE END


![关于”PostgreSQL错误:关系[表]不存在“问题的原因和解决方案-yiteyi-C++库](https://www.yiteyi.com/wp-content/themes/zibll/img/thumbnail.svg)



